The Patterns and Nonlinear Functions Quiz is an interactive assessment tool designed to evaluate students’ understanding of patterns and nonlinear functions. This quiz delves into the intricacies of mathematical functions, exploring their patterns and characteristics, and challenging students to apply their knowledge to solve real-world problems.
This quiz covers a wide range of topics, including the identification and analysis of patterns in functions, the definition and properties of nonlinear functions, and the use of graphing, tables, and other tools to analyze patterns in nonlinear functions.
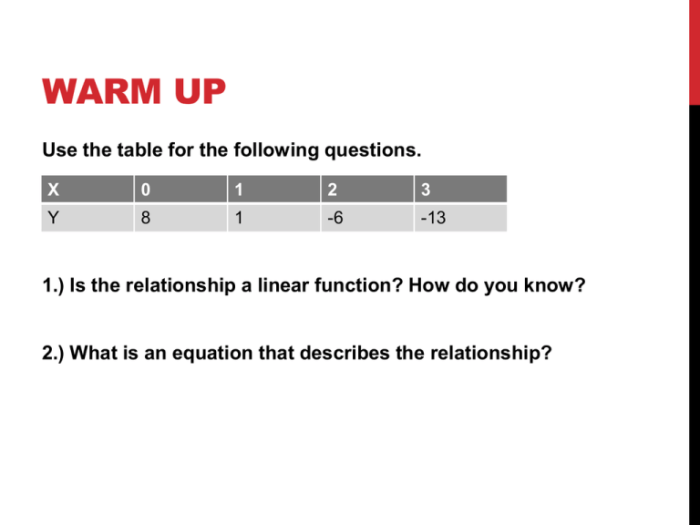
Patterns and Nonlinear Functions
Mathematical functions often exhibit patterns that can provide valuable insights into their behavior. Identifying and analyzing these patterns can help us understand the function’s properties, predict its behavior, and apply it in real-world scenarios.
Patterns in Functions
Patterns in functions refer to regularities or relationships that exist within the function’s output values. These patterns can manifest in various forms, such as:
- Linear patterns: Functions that produce a straight line when graphed.
- Quadratic patterns: Functions that produce a parabola when graphed.
- Exponential patterns: Functions that produce a curve that increases or decreases rapidly.
- Periodic patterns: Functions that repeat a pattern over regular intervals.
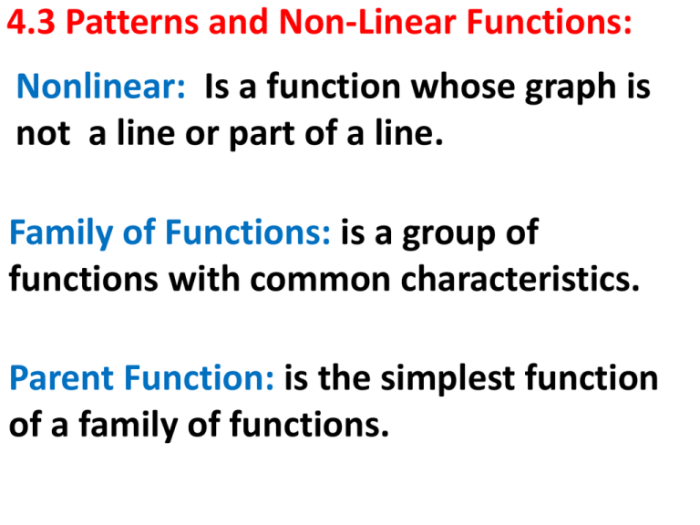
Nonlinear Functions
Nonlinear functions are functions that do not produce a straight line when graphed. They exhibit complex relationships between their input and output values, and their behavior can be more challenging to predict than linear functions.
Common examples of nonlinear functions include:
- Polynomials: Functions with terms involving powers of the independent variable.
- Exponentials: Functions that involve raising a constant to the power of the independent variable.
- Logarithms: Functions that involve taking the logarithm of the independent variable.
Analyzing Patterns in Nonlinear Functions
Analyzing patterns in nonlinear functions requires a combination of algebraic and graphical techniques:
- Graphing: Plotting the function’s output values for different input values can reveal patterns in the graph’s shape.
- Tables: Creating a table of input-output pairs can help identify patterns in the function’s values.
- Algebraic analysis: Using algebraic methods, such as factoring and completing the square, can simplify the function and make patterns more apparent.
Quiz Design
To design an effective quiz on patterns and nonlinear functions, consider the following guidelines:
- Clear instructions: Provide clear instructions and examples to ensure students understand the expectations.
- Variety of question types: Include multiple choice, short answer, graphing, and problem-solving questions to assess different skills.
- Appropriate difficulty levels: Ensure the questions cover a range of difficulty levels to accommodate students with varying abilities.
Example Quiz Questions, Patterns and nonlinear functions quiz
| Question Type | Example Question |
|---|---|
| Multiple Choice | Which of the following is the equation of a quadratic function with a vertex at (2, 3)? |
| Short Answer | Describe the pattern in the output values of the function f(x) = 2^x. |
| Graphing | Graph the function g(x) = x^3
|
| Problem-Solving | A company’s profit is given by the function P(x) =
|
Scoring and Evaluation
Scoring and evaluating the quiz should be based on the following:
- Accuracy: Award points for correct answers.
- Completeness: Ensure students provide sufficient details in their responses.
- Clarity: Assess the clarity and organization of students’ explanations.
Detailed FAQs: Patterns And Nonlinear Functions Quiz
What is the purpose of the Patterns and Nonlinear Functions Quiz?
The Patterns and Nonlinear Functions Quiz is designed to assess students’ understanding of patterns and nonlinear functions, and to foster critical thinking and problem-solving skills.
What topics does the quiz cover?
The quiz covers a wide range of topics, including the identification and analysis of patterns in functions, the definition and properties of nonlinear functions, and the use of graphing, tables, and other tools to analyze patterns in nonlinear functions.
How is the quiz scored?
The quiz is scored based on the accuracy of students’ responses to the questions. A scoring rubric or guidelines for assessing student responses is provided to ensure consistency and fairness in grading.